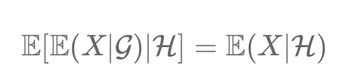
The Tower Property in Simple Terms

The Tower Property, also known as the Law of Iterated Expectation, is a fundamental concept in probability theory, particularly when working with conditional expectations and \( \sigma \)-algebras.

\[ \mathbb{E}[\mathbb{E}(X|\mathcal{G})|\mathcal{H}] = \mathbb{E}(X|\mathcal{H}) \]

This property applies under the condition that \( \mathcal{H} \) is a sub-\( \sigma \)-algebra of \( \mathcal{G} \).¹

X is a random variable.

\( \mathcal{G} \) and \( \mathcal{H} \) are \( \sigma \)-algebras with \( \mathcal{H} \) being a sub-\( \sigma \)-algebra of \( \mathcal{G} \) (meaning every set in \( \mathcal{H} \) is also in \( \mathcal{G} \)).

\( \mathbb{E}[\mathbb{E}(X|\mathcal{G})|\mathcal{H}] \): This expression first calculates the conditional expectation of X given \( \mathcal{G} \), and then it calculates the conditional expectation of that result given \( \mathcal{H} \).

\( \mathbb{E}(X|\mathcal{H}) \): This is the direct calculation of the conditional expectation of X given \( \mathcal{H} \).

The Tower Property states that if you first condition on a 'broader' \( \sigma \)-algebra (\( \mathcal{G} \)) and then on a 'narrower' one (\( \mathcal{H} \)), it is equivalent to directly conditioning on the narrower \( \sigma \)-algebra (\( \mathcal{H} \)).

Intuitively, it means that when you refine your conditioning from a broader context to a more specific one, the overall expectation aligns with conditioning directly on the more specific context.

Example: Consider a two-stage investment horizon: mid-year and end-of-year.

Let \( \mathcal{H} \) be the \( \sigma \)-algebra representing information available up to mid-year. Let \( \mathcal{G} \) be a larger \( \sigma \)-algebra representing additional information available by the end-of-year, which includes \( \mathcal{H} \).

X represents the total return of the portfolio at the end of the year.

\( \mathbb{E}(X|\mathcal{G}) \): This is the expected return of the portfolio at the end of the year, calculated with all the information available by then.

\( \mathbb{E}[\mathbb{E}(X|\mathcal{G})|\mathcal{H}] \): Here, you first compute the expected return at the end of the year (using all year-end information) and then adjust this expectation to what would be expected at mid-year, using only the information available at mid-year.

\( \mathbb{E}(X|\mathcal{H}) \): This is the direct expected return of the portfolio at the end of the year, but evaluated at mid-year using only mid-year information.

The Tower Property,

\[ \mathbb{E}[\mathbb{E}(X|\mathcal{G})|\mathcal{H}] = \mathbb{E}(X|\mathcal{H}) \]

tells us that the expectation of the year-end return, when adjusted at mid-year, is the same whether we account for the additional information that will be available by the end of the year or not.

In summary, the Tower Property illustrates that when evaluating expectations with respect to a certain level of information (\( \mathcal{H} \)), any further details available in a larger \( \sigma \)-algebra (\( \mathcal{G} \)) do not impact this expectation. It highlights the sufficiency of the current level of information (\( \mathcal{H} \)) for the purpose of calculating expectations at that stage.

This property allows simplifying complex conditional expectations by breaking them down into stages, aligning with the natural filtration of information.

Notes:

¹ A sigma algebra (\( \sigma \)-algebra) is a collection of subsets of a set (X) that includes the empty set, is closed under complementation, and is closed under countable unions. It's used in measure theory to define measures like probability.