The Merton model is recognized as a fundamental building block of what are known as structural models to assess the probability of default in credit risk analysis. This model’s approach to conceptualizing a company’s equity as a call option on its assets has paved the way for the development and refinement of structural models in the financial sector.
According to this model, a corporation's asset value at any time t (At) comprises its stock equity (Et) and zero-coupon debt (Dt) with a face amount of K, maturing at a future time T, where T is greater than t. This relationship forms the equation At = Et + Dt. At the debt's maturity (T), the firm's equity value (Et) is determined as the maximum of At - K or zero. If At exceeds K, equity holders benefit from the difference; otherwise, the equity becomes worthless, leading to default.
The Merton model uses the Black-Scholes formula to value this 'call option' on the firm's assets, where:
E= At * N(d1) - K * e^(-r(T-t)) * N(d2)
- E is the equity value.
- N() is the cumulative distribution function of the standard normal distribution, and d1 and d2 are functions of asset value, debt level, risk-free interest rate r, and asset volatility sigma.
However, the Merton model, despite being a pioneering structural credit risk model, has encountered criticisms. Its heavy reliance on market data for asset value and volatility estimates limits its applicability to private companies or those with less liquid stocks.
The model's assumptions of frictionless markets and the firm's inability to renegotiate debt do not always align with real-world conditions. It tends to underestimate short-term default risks and may not provide early warning signs of financial distress.
These critiques have spurred the development of alternative models, such as reduced form models. These models treat default as a sudden, unpredictable event, influenced by external factors, and often employ stochastic methods like the Poisson process for default modeling.
Unlike structural models, reduced form models do not rely on a firm's asset and liability structure and are adaptable to a broader range of borrowers. They have become particularly useful in pricing credit derivatives like credit default swaps.
While the Merton model introduced a significant advance in credit risk modeling by applying option pricing theory to firm equity, its limitations have led to the emergence of other models, notably reduced form models. These newer models overcome some of the Merton model's weaknesses, particularly in scenarios where market data is scarce or unreliable, providing alternative means to comprehend and quantify credit risk.
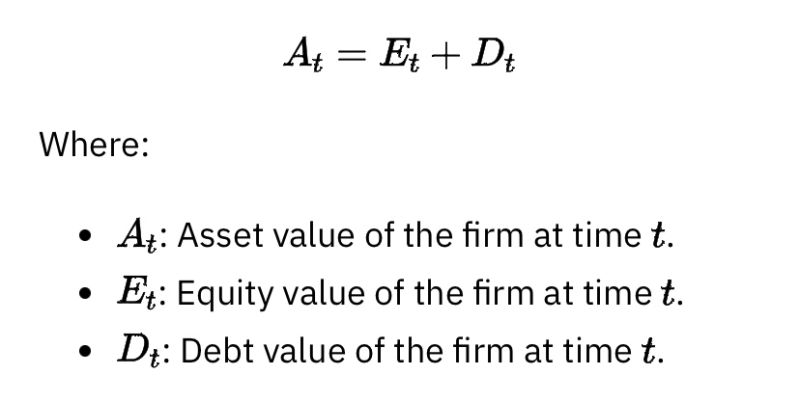

Écrire commentaire