Perpetuities in Finance Simply Explained

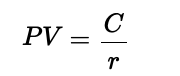
A perpetuity is a financial instrument that provides cash flows indefinitely. The present value (PV) of a perpetuity is:

\[ PV = \frac{C}{r} \]

where \( C \) is the cash flow and \( r \) is the discount rate.

Why Are Perpetuities Useful?

1. Stock Valuation: Preferred stocks often pay fixed dividends forever.

2. Real Estate: Properties with stable rental income are valued using perpetuity models.

3. Bond Pricing: Consol bonds pay interest perpetually.

4. Corporate Valuation: Terminal values in discounted cash flow (DCF) models often use perpetuities.

Link to Geometric Series

A perpetuity is an infinite sum of discounted cash flows:

\[ PV = \frac{C}{(1 + r)} + \frac{C}{(1 + r)^2} + \frac{C}{(1 + r)^3} + \dots \]

This is a geometric series with first term \( a = \frac{C}{(1 + r)} \) and common ratio \( q = \frac{1}{(1 + r)} \). The formula for an infinite geometric series gives:

\[ PV = \frac{C}{r} \]

Growing Perpetuity

If cash flows grow at a constant rate \( g \), the present value formula is:

\[ PV = \frac{C}{r - g} \]

where \( g \) is the growth rate.

Why Do We Subtract \( g \)?

A growing perpetuity follows:

\[ PV = \frac{C}{(1 + r)} + \frac{C(1 + g)}{(1 + r)^2} + \frac{C(1 + g)^2}{(1 + r)^3} + \dots \]

This geometric series has first term \( \frac{C}{(1 + r)} \) and common ratio \( \frac{(1 + g)}{(1 + r)} \), which simplifies to:

\[ PV = \frac{C}{r - g} \]

If \( g \) is close to \( r \), \( PV \) becomes very large. If \( g = r \), the formula breaks down, implying infinite value.

Example

A stock pays $2 dividends, growing at 3% per year. If the required return is 7%, its value is:

\[ P = \frac{2}{0.07 - 0.03} = 50 \]

This is higher than a standard perpetuity, since future cash flows increase.