The Black Scholes model is widely known for calculating the theoretical value of European style options, assuming that stock prices follow a lognormal distribution. Within this model, a key component is d₁, a term that captures several important aspects of option pricing. Understanding d₁ is crucial for gaining insight into how options behave and are valued.
Formula Breakdown:
The d₁ term is defined as follows:
d₁ = (ln(S₀/X) + (r + σ²/2) T) / (σ √T)
Where:
S₀: Current stock price
X: Option strike price
T: Time to expiration (in years)
r: Risk-free interest rate (annualized)
σ: Volatility of the stock (annualized)
Let’s break down this formula to understand its key components and how they influence the option's price.
Key Components of d₁:
1. Natural Logarithm of the Stock to Strike Ratio: ln(S₀/X)
This part of d₁ measures the relative position of the stock price to the strike price. If the stock price (S₀) is greater than the strike price (X), then the natural logarithm (ln(S₀/X)) is positive, suggesting that the option is "in the money." Conversely, if S₀ is less than X, the term is negative, indicating that the option is "out of the money." The natural logarithm helps transform this ratio into a return representation, considering the compounding effects over time.
2. Risk-free Rate Compensation: rT
This component captures the time value of money. The risk-free rate (r) reflects the return on an entirely risk-free investment over the time to expiration (T). This term adjusts for the fact that receiving money in the future is worth less than receiving it today, taking into account the opportunity cost of capital over the option’s lifespan.
3. Volatility Adjustment: (σ²/2)T
This term accounts for the volatility of the stock. Volatility (σ) measures the stock price's variability, and a higher volatility means more uncertainty and potential for price swings. The factor (σ² / 2) arises from the stochastic nature of stock price evolution (specifically, geometric Brownian motion), adjusting for the skewness in the distribution of returns.
4. Denominator Volatility Adjusted for Time: σ√T
The denominator standardizes d₁ by considering both the stock’s volatility (σ) and the time to expiration (T). The σ√T term captures how uncertainty (volatility) scales with the square root of time, reflecting that as time increases, the potential variability in the stock price also increases.
Interpreting d₁ in Context
In essence, d₁ can be interpreted as the standardized distance between the current stock price and the strike price, adjusted for the time value of money and expected volatility. It quantifies how "close" the stock price is to being in or out of the money when considering all the relevant financial parameters.
N(d₁): The Option’s Delta
A critical aspect of d₁ is its relationship to N(d₁), the cumulative probability under the standard normal distribution. This value, N(d₁), plays a vital role in option pricing because it represents the delta of a European call option.
What is Delta?
Delta measures how sensitive the option price is to small changes in the underlying stock price. For example, a delta of 0.50 indicates that for a $1 change in the stock price, the option price is expected to change by $0.50.
In practical terms, N(d₁) tells you how much the value of the option will change in response to a small movement in the stock price.
Common Misconception: N(d₁) vs. N(d₂)
A common misconception is to interpret N(d₁) as the probability of the option expiring "in the money." However, this is not correct—N(d₂) actually serves this role. N(d₂) represents the risk neutral probability that the option will end up in the money at expiration.
While N(d₁) is related to the likelihood of the option expiring in the money, its primary function is to represent the option’s delta, which is the sensitivity of the option's price to changes in the underlying stock price. The delta, N(d₁), not only gives an indication of how much the option’s price will change for a $1 move in the stock but also reflects the hedge ratio, i.e., how much of the stock you would need to hold to be delta neutral.
Why is N(d₁) Important?
N(d₁) ranges between 0 and 1, making it effectively a probability like value. A higher N(d₁) means that the option is more sensitive to the stock price movements and has a higher delta.
N(d₁) also reflects the "moneyness" of the option. When d₁ is high, meaning that the stock price is significantly greater than the strike price, N(d₁) approaches 1. Conversely, when d₁ is low, N(d₁) approaches 0, indicating that the option is far out oft the money.
In summary, d₁ encapsulates multiple factors of option pricing, such as stock price, strike price, volatility, time, and interest rates. Meanwhile, N(d₁) serves as the delta, providing insights into the option’s sensitivity to price changes and how to hedge effectively. N(d₂), on the other hand, represents the probability of the option ending in the money, clarifying the distinct roles that each term plays in the Black-Scholes model.
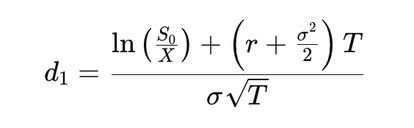
Écrire commentaire