The Black-Scholes model is one of the most influential frameworks for pricing European options. At its core, the model incorporates two key probabilities: \( N(d_1) \) and \( N(d_2) \). Although both are cumulative distribution functions of the standard normal distribution, they serve distinct purposes in determining the value of a European call or put option.
While \( N(d_2) \) reflects the likelihood of an option expiring in the money (ITM) under the risk-neutral framework, \( N(d_1) \) adjusts for the expected stock price if the option is exercised.
Understanding these two terms is essential for interpreting the mechanics of the Black-Scholes formula and for appreciating the interplay between intrinsic value and time value in options
pricing.
This article breaks down the roles of \( N(d_1) \) and \( N(d_2) \) with a focus on their differences, their contributions to the option value, and why \( N(d_1) > N(d_2) \) ensures no-arbitrage pricing.
Key Components of the Black-Scholes Formula
The Black-Scholes formula for a European call option is:
\[ C = S \cdot N(d_1) - X \cdot e^{-rT} \cdot N(d_2), \]
where:
- \( C \): Price of the call option
- \( S \): Current stock price
- \( X \): Strike price
- \( r \): Risk-free interest rate
- \( T \): Time to maturity
- \( N(d_1) \), \( N(d_2) \): Standard normal cumulative distribution functions
The terms \( N(d_1) \) and \( N(d_2) \) are integral to the valuation:
- \( N(d_2) \): The risk-neutral probability of the option expiring ITM (\( S_T > X \))
- \( N(d_1) \): A risk-adjusted measure accounting for the expected stock price if the option is exercised
Intuition Behind \( N(d_1) \) and \( N(d_2) \)
To understand the roles of \( N(d_1) \) and \( N(d_2) \), consider the two primary components of the Black-Scholes formula:
- \( S \cdot N(d_1) \): Represents the present value of the stock price, adjusted for the likelihood of the option being exercised. \( N(d_1) \) incorporates both the probability of exercise and the conditional expectation of the stock price if the option is ITM.
-
\( X \cdot e^{-rT} \cdot N(d_2) \): Represents the discounted value of the strike price, adjusted for the probability of the option being exercised (\( N(d_2) \)).
The difference between these two terms determines the option value. Since \( N(d_1) > N(d_2) \), the term \( S \cdot N(d_1) \) is always greater than \( X \cdot e^{-rT} \cdot N(d_2) \), ensuring the option value is non-negative.
Why \( N(d_1) > N(d_2) \) Matters
The inequality \( N(d_1) > N(d_2) \) reflects the fact that \( N(d_1) \) considers the conditional expectation of the stock price, while \( N(d_2) \) accounts only for the probability of the option being ITM. This adjustment ensures that the value of the call option not only reflects the likelihood of exercise but also the potential payoff from holding the option.
The distinction between \( N(d_1) \) and \( N(d_2) \) highlights the balance between intrinsic value (driven by the difference between the stock price and the strike price) and time value (the potential for the stock price to move further ITM before expiration).
The Black-Scholes model provides a sophisticated yet practical framework for pricing European options. Central to its success are the roles of \( N(d_1) \) and \( N(d_2) \), which together capture the probabilities and expectations required for fair pricing under a risk-neutral measure. By understanding these terms, traders and analysts can better interpret option values and devise effective hedging strategies.

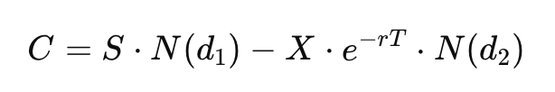
Write a comment